Difference between revisions of "Iterate of exponential"
| Line 25: | Line 25: | ||
This representation defines the $n$th iterate for any complex number $n$ of iterations. |
This representation defines the $n$th iterate for any complex number $n$ of iterations. |
||
| + | Methods for the evaluation are described in 2009 by D.Kouznetsov in [[Mathematics of Computation]] |
||
| + | <ref> |
||
| + | http://www.ams.org/mcom/2009-78-267/S0025-5718-09-02188-7/home.html<br> |
||
| + | http://www.ils.uec.ac.jp/~dima/PAPERS/2009analuxpRepri.pdf<br> |
||
| + | http://mizugadro.mydns.jp/PAPERS/2009analuxpRepri.pdf |
||
| + | D. Kouznetsov. Solution of $F(x+1)=\exp(F(x))$ in complex $z$-plane. 78, (2009), 1647-1670 |
||
| + | </ref>, and the efficient [[C++]] complex double implementation are described in 2010 in [[Vladivavkaz mathematical Journal]] (in Russian); the English version is also loaded |
||
| + | <ref> |
||
| + | http://www.ils.uec.ac.jp.jp/~dima/PAPERS/2009vladie.pdf (English) <br> |
||
| + | http://mizugadro.mydns.jp/PAPERS/2010vladie.pdf (English) <br> |
||
| + | http://mizugadro.mydns.jp/PAPERS/2009vladir.pdf (Russian version) <br> |
||
| + | D.Kouznetsov. Superexponential as special function. Vladikavkaz Mathematical Journal, 2010, v.12, issue 2, p.31-45. |
||
| + | </ref> |
||
| + | |||
| + | </ref>. |
||
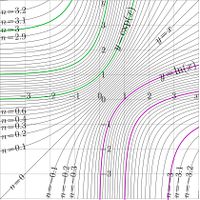
Complex maps of the $n$th iteration of exponential is shown in figures at right with |
Complex maps of the $n$th iteration of exponential is shown in figures at right with |
||
Revision as of 10:35, 27 July 2013
Iteration of exponential (or Iteration of rxponent) is function $f(z)=\exp^n(z)$, where upper superscript indicates the number of iteration.
In TORI, the number in superscript after a name of any function denotes the number of iteration. This notation is neither new, nor original; Walter Bergweiler had used it in century 20 [1].
This article is under construction. Wait for few days before to use it. 01:37, 27 July 2013 (JST)
The most often are the first iteration of exponent, $n=1$; $\exp^1=\exp$
and the minus first iteration, $n=-1$; $\exp^{-1} = \ln$.
Less often they appear with $n = \pm 2$; $\exp^2(z)=\exp(\exp(z))$, and $\exp^{-2}(z)=\ln(\ln(z))$. Other values of number of iteration are not usual, and until 2008, there was no regular way to evaluate iteration of exponential for any non–integer number $n$ of iteration. However, with tetration tet, that is superfunction of exponent, and Arctetration ate, that is Abel function of exponent, the $n$th iteration can be expressed as follows:
$\exp^n(z)=\mathrm{tet}(n+\mathrm{ate}(z))$
This representation defines the $n$th iterate for any complex number $n$ of iterations. Methods for the evaluation are described in 2009 by D.Kouznetsov in Mathematics of Computation [2], and the efficient C++ complex double implementation are described in 2010 in Vladivavkaz mathematical Journal (in Russian); the English version is also loaded [3]
</ref>.
Complex maps of the $n$th iteration of exponential is shown in figures at right with lines $u=\Re(f(x+\mathrm i y))$ and lines $v=\Im(f(x+\mathrm i y))$ for various values $n$.
References
- ↑ http://www.ams.org/journals/bull/1993-29-02/S0273-0979-1993-00432-4/S0273-0979-1993-00432-4.pdf Walter Bergweiler. Iteration of meromorphic functions. Bull. Amer. Math. Soc. 29 (1993), 151-188.
- ↑
http://www.ams.org/mcom/2009-78-267/S0025-5718-09-02188-7/home.html
http://www.ils.uec.ac.jp/~dima/PAPERS/2009analuxpRepri.pdf
http://mizugadro.mydns.jp/PAPERS/2009analuxpRepri.pdf D. Kouznetsov. Solution of $F(x+1)=\exp(F(x))$ in complex $z$-plane. 78, (2009), 1647-1670 - ↑
http://www.ils.uec.ac.jp.jp/~dima/PAPERS/2009vladie.pdf (English)
http://mizugadro.mydns.jp/PAPERS/2010vladie.pdf (English)
http://mizugadro.mydns.jp/PAPERS/2009vladir.pdf (Russian version)
D.Kouznetsov. Superexponential as special function. Vladikavkaz Mathematical Journal, 2010, v.12, issue 2, p.31-45.
http://www.ams.org/journals/bull/1993-29-02/S0273-0979-1993-00432-4/S0273-0979-1993-00432-4.pdf Walter Bergweiler. Iteration of meromorphic functions. Bull. Amer. Math. Soc. 29 (1993), 151-188.
http://www.ams.org/mcom/2009-78-267/S0025-5718-09-02188-7/home.html
http://www.ils.uec.ac.jp/~dima/PAPERS/2009analuxpRepri.pdf
http://mizugadro.mydns.jp/PAPERS/2009analuxpRepri.pdf
D. Kouznetsov. Solution of $F(x+1)=\exp(F(x))$ in complex $z$-plane. 78, (2009), 1647-1670
Keywords
Abel function, Arctetration, Exponent, Iteration, Superfunction, Tetration,,,,,,,