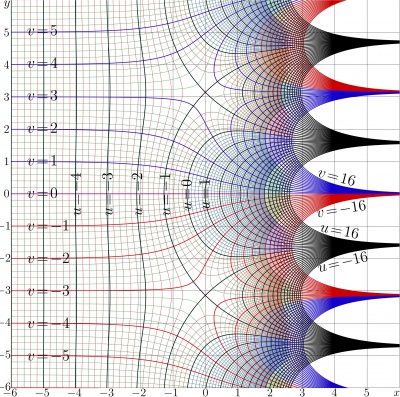
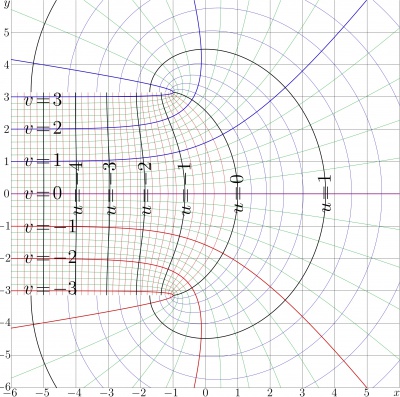
Trappmann function
Trappmann function is defined with
\(~\mathrm{Tra}(z)=\mathrm e^z +z~\)
From definition it follows, that it is entire elementary function. The Trappmann function is example of holomorphic function without fixed points, suggested in year 2011 by Henryk Trappmann [1]. The absence of fixed points had been considered as a serious obstacle at the building-up its superfunction, the Abel function and the non–integer iterates of function Tra.
According to the general statement by [2], it is possible to construct at least one superfunction for any holomorphic function. Therefore, the consideration of the Trappmann function as transfer function is important.
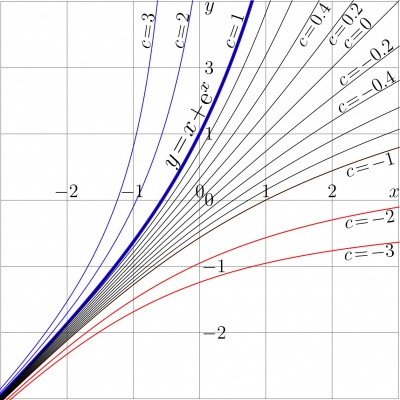
Complex map of the Trappmann function is shown at the top figure. The Seconf figure shows the map of its inverse function ArcTra, and the Third (last) figure shows the explicit plot \(y=\mathrm{Tra}(x)\) in comparison to various iterates of this function.
Contents
About the names
The name of function \(\mathrm{Tra}\) or \(\mathrm{tra}\) is chosen after the last name of Henryk Trappmann [1], because it begins with letter \(T\), often used to denote the Transfer function. The question, wether to capitalize the first letter in the name of the function or not, is still under investigation.
Name ArcTra is suggested for the inverse function, id est, the minus first iteration; \(\mathrm{ArcTra}=\mathrm{Tra}^{-1}\)
Name SuTra is suggested for the superfunction and name AuTra is suggested for the Abel function.
In this article, the properties of function Tra is described, and properties of SuTra and AuTra are mentioned.
While, the capitalisation of the first character of the name of the function has no specific meaning, and there is no difference between \(\mathrm {Tra}\) and \(\mathrm {Tra}\). The reason to use the capitalisation is mainly technical, than mathematical: the names of all articles in mediawiki (TORI uses the mediawiki platform) are capitalised by default, while writing formulas, typing name with capital letter requires to press an additional key. In the similar way, in the Mathematica software, all the names of the implemented special functions begin with capital letter. In this sense, \(\mathrm{Tra}=\mathrm{tra}\). As for the super functions, the capitalisation (for example, \(\mathrm{SuTra}\)) is used to separate the prefix "Su" (that indicate, that this is superfunction) from the name of the transfer function.
Properties
Asymptotically, SuZex behaves like a linear function while the real part of the argument is negative, and as exponential, while the real part of the argument is positive.
SuZex has a countable set of saddle points, \(\mathrm 2 i \pi (n+1/2)\) for integer values of \(n\).
Inverse function
Inverse function ArcTra, id est, \(~\mathrm{ArcTra}=\mathrm{Tra}^{-1}~\) can be expressed through the WrightOmega function as well as through the Tania function as follows:
\(\mathrm{ArcTra}(z)=\) \(z-\mathrm{Tania}(z\!-\!1)=\) \( z-\mathrm{WrightOmega}(z)\)
The complex map of the ArcTra function is shown in Figure 2.
Exponential modification, superfunction and the Abel function
Idea by Henryk is that it the fixed point does not exist, them we should create it, modifying the Transfer function. The simple modification is described in this section. Let the Transfer function \(T\) be defined with
(1) \( ~ ~ ~ T(z)=\mathrm{tra}(z)=\mathrm e^z+z\)
Let \(~F\!=\!\mathrm{hen}~\) be its superfunction and \(~G\!=\!F^{-1}\!=\!\mathrm{ahe}~\) be its superfunction and the Abel function. The superfunction should satisfy the transfer equation
(2) \(~ ~ ~ T\!\Big(F(x)\Big) = F(z\!+\!1)\)
and the Abel function should satisfy the Abel equation
(3) \(~ ~ ~ G\!\Big(T(x)\Big) = G(z) + 1\)
Search the solution for \(F\) in the following form:
(4) \( ~ ~ ~ F(z)=\ln\!\Big(h(z)\Big)\)
where \(h\) is some holomorphic function. The reason for this representation is a hope, that the corresponding transfer equation for \(h\) will be simpler, than the initial transfer equation Form (4), function \(h\) can be expressed as follows:
(5) \(~ ~ ~ h(z)=\exp\!\Big(F(z)\Big)\)
Substituting representation (2) into the transfer equation (2) gives
(6) \( ~ ~ ~ T\!\Big(\ln\big(h(z)\big)\Big)=\ln\!\Big(h(z\!+\!1)\Big)\)
Using equation (1), equation (6) gives:
(7) \( ~ ~ ~ h(z\!+\!1) =\) \( \exp\!\Big( T\!\Big(\ln\big(h(z)\big)\Big) \Big)=\) \( \exp\!\Big( h(z) +\ln\big(h(z)\big)\Big) =\) \( h(z) \ln\!\Big(h(z)\Big)=\mathrm{zex}\!\Big( h(z) \Big)\)
where
(8) \( ~ ~ ~ \mathrm{zex}(z)=z\, \exp(z)\)
Properties of function zex are already described, and SuZex is its superfunction, id est, \(h=\mathrm{SuZex}\) is solution of equation
(9) \( ~ ~ ~ h(z\!+\!1) = \mathrm{zex}\!\Big( h(z) \Big)\)
Therefore, solution of the transfer equation (2) for \(T=\mathrm{Tra}\) is
(10) \( ~ ~ ~ \mathrm{SuTra}(z) = \ln\!\Big(\mathrm{SuZex}(z) \Big)\)
and the corresponding Abel function \(G\) can be expressed with
(11) \( ~ ~ ~ \mathrm{AuTra}(z)= \mathrm{AuZex}\!\Big( \exp(z) \Big)\)
at least for \(|\Im(z)|\le \pi\).
The efficient algorithms for evaluation of SuZex and AuZex are already loaded; so, the evaluation of the superfunction and the Abel function for transfer function Tra by (1) should not cause any difficulties. With these functions, the non-integer iterates of the Trappmann function are evaluated to plot figure 1.
In 2013, the direct implementation for function SuTra is suggested [3] The numeric implementation is loaded as sutran.cin. The direct representation looks simpler, a little but faster and provide a little bit better precision due to reduction of the rounding errors at evaluation of exponential and logarithm of huge values.
It is not yet clear, which notation is better, with capitalisation or just lowercase letters. For reading, the capitalisation seems to be better. On the other hand, the Macintosh operational system easy gets confused with capitalisation; and for the authomatic handling with robots, the lowercase notation seem to be better.
Inverse function and non-integer iterate
With superfunction \(F\) and the Abel function \(G\) by (10) and (11), the inverse function of \(\mathrm{tra}\) can be expressed also in the following form
(12) \( ~ ~ ~ \mathrm{tra}^{-1}(z)=F\!\Big(-1+G(z)\Big)\)
This expression corresponds to the curve with \(c\!=\!-1\) in Figure 1. In general, the \(c\)th iteration can be expressed as usually,
(13) \( ~ ~ ~ \mathrm{tra}^{n}(z)=F\!\Big(n+G(z)\Big)\)
The function without fixed point does not break the general concept; it seems, the non-integer iterates can be defined for any holomorphic transfer function.
References
- ↑ 1.0 1.1 http://math.eretrandre.org/publications.html Publication List// Henryk Trappmann.
- ↑ http://www.springerlink.com/content/qt31671237421111/fulltext.pdf?page=1 D.Kouznetsov, H.Trappmann. Superfunctions and square root of factorial. Moscow University Physics Bulletin, 2010, v.65, No.1, p.6-12.
- ↑
http://www.m-hikari.com/ams/ams-2013/ams-129-132-2013/kouznetsovAMS129-132-2013.pdf
http://mizugadro.mydns.jp/PAPERS/2013hikari.pdf D.Kouznetsov. Entire function with logarithmic asymptotic. Applied Mathematical Sciences, 2013, v.7, No.131, p.6527-6541.
Keywords
Abel function, Abel equation, AuZex Fixed point, Henryk Trappmann, Iteration, LambertW, Transfer function, Transfer equation, Superfunction, SuZex, Zex,