Difference between revisions of "Theorem on increment of tetration"
| Line 57: | Line 57: | ||
https://www.morebooks.de/store/gb/book/superfunctions/isbn/978-620-2-67286-3 |
https://www.morebooks.de/store/gb/book/superfunctions/isbn/978-620-2-67286-3 |
||
Dmitrii Kouznetsov. [[Superfunctions]]. Lambert Academic Publishing, 2020. |
Dmitrii Kouznetsov. [[Superfunctions]]. Lambert Academic Publishing, 2020. |
||
| + | ==Keywords== |
||
| + | [[Asymptotic analysis]], |
||
| + | [[Filog]], |
||
| + | [[Fixed point]], |
||
| + | [[Growing factor]], |
||
| + | [[Increment]], |
||
| + | [[Kneser Expansion]], |
||
| + | [[Superfunction]], |
||
| + | [[Tetration]] |
||
| + | |||
| + | [[Category:Asymptotic analysis]] |
||
| + | [[Category:English]] |
||
[[Category:Kneser Expansion]] |
[[Category:Kneser Expansion]] |
||
[[Category:Superfunction]] |
[[Category:Superfunction]] |
||
Revision as of 13:24, 12 August 2020
Theorem on increment of tetration is statement about asymptotic behavior of solution of the Transfer equation with exponential transfer functions. It applies not only to tetration, but also to other superexponentials.
Statement
Let \(F\) be solution of equation
\(F(z\!+\!1)=\exp\big(\beta F(z)\big)\)
for some \(\beta>0\).
Let \(L\) be the fixed point, id est, \(\exp(\beta L)=L\)
Let \(F(z)=L+\varepsilon+O(\varepsilon^2) \)
where \(\varepsilon = \exp(kz) \) for some increment \(k\).
Let \(~ K\!=\!\exp(k)\)
Then
\( \Im(K) = \Im(k) \)
Applications
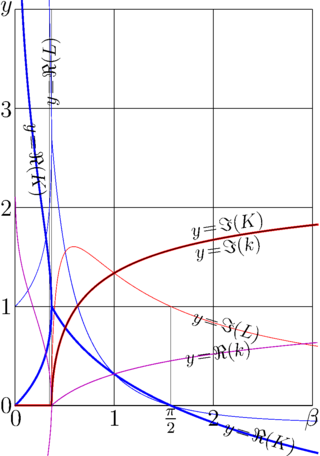 Fig.1. Asymptoric parameters of Tetration versus \(\beta\)
Fig.1. Asymptoric parameters of Tetration versus \(\beta\)
Fig.1 shows the asymptotic parameters of tetration to base \(\ln(\beta)\):
Real and imaginary parts of the fixed points
\(L=L_1=\) Filog\((\beta)\)
and
\(L=L_1=\) Filog\((\beta^*)^*\)
Real and imaginary parts of the asymptotic growing factor
\(K= \beta L\)
Real and imaginary parts of the asymptotic increment
\(k=\ln(K) \)
For \(\beta < 1/\mathrm e\), the two fixed points are shown; and the two values of the corresponding growing factor and two values of the corresponding increment are drown.
For real positive \(\beta\),
The imaginary parts of \(K\) and \(k\) coincide.
References
https://www.morebooks.de/store/gb/book/superfunctions/isbn/978-620-2-67286-3 Dmitrii Kouznetsov. Superfunctions. Lambert Academic Publishing, 2020.
Keywords
Asymptotic analysis, Filog, Fixed point, Growing factor, Increment, Kneser Expansion, Superfunction, Tetration