Iterate of exponential

$u+\mathrm i v=\exp^1(x+\mathrm i y)=\exp(x+\mathrm i y)$

$u+\mathrm i v=\exp^{0.9}(x+\mathrm i y)$

Iteration of exponential (or Iteration of rxponent) is function $f(z)=\exp^n(z)$, where upper superscript indicates the number of iteration.

This article is under construction. Wait for few days before to use it. 01:37, 27 July 2013 (JST)

The most often are the first iteration of exponent, $n=1$; $\exp^1=\exp$

and the minus first iteration, $n=-1$; $\exp^{-1} = \ln$.

Less often they appear with $n\ne \pm 2$; $\exp^2(z)=\exp(\exp(z))$, and $\exp^{-2}(z)=\ln(\ln(z))$. Other values of number of iteration are not usual.

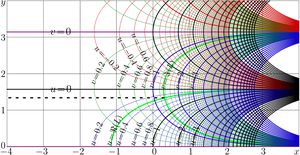
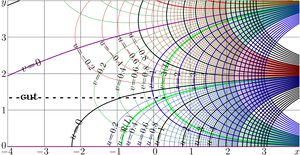
Until 2008, there was no regular way to evaluate iteration of exponential for non–integer number $n$ of iteration. However, with tetration tet, that is superfunction of exponent, and Arctetration ate, that is Abel function of exponent, the $n$th iteration can be expressed as follows:

$\exp^n(z)=\mathrm{tet}(n+\mathrm{ate}(z))$

This representation defines the $n$th iterate for any complex number $n$ of iterations.

Complex maps of the $n$th iteration of exponential is shown in figures at right with lines $u=\Re(f(x+\mathrm i y))$ and lines $v=\Im(f(x+\mathrm i y))$ for various values $n$.

Keywords

Abel function, Arctetration, Exponent, Iteration, Superfunction, Tetration,,,,,,,