Difference between revisions of "BesselY0"
m (Text replacement - "\$([^\$]+)\$" to "\\(\1\\)") |
|||
| Line 1: | Line 1: | ||
[[File:BesselY0J0J1plotT060.png|500px|thumb| |
[[File:BesselY0J0J1plotT060.png|500px|thumb| |
||
[[Explicit plot]] of [[BesselY0]] (red), [[BesselJ1]] (green) and [[BesselJ0]] (blue)]] |
[[Explicit plot]] of [[BesselY0]] (red), [[BesselJ1]] (green) and [[BesselJ0]] (blue)]] |
||
| − | [[File:Bessely0mapT064.png|500px|thumb|[[Complex map]] |
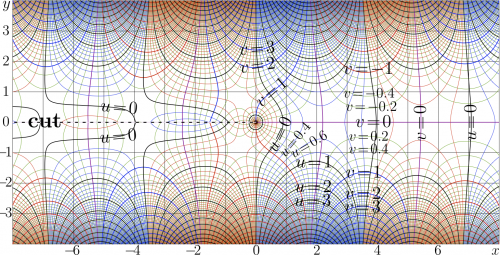
+ | [[File:Bessely0mapT064.png|500px|thumb|[[Complex map]] \(u+\mathrm i v=Y_0(x+\mathrm i y)\) ]] |
| − | '''BesselY0''', called also [[Neumann function]], and also |
+ | '''BesselY0''', called also [[Neumann function]], and also \(\mathrm{BesselY}_0\) or simply \(Y_0\) is |
kind of [[Bessel function]] |
kind of [[Bessel function]] |
||
(or [[Cylindric function]]), |
(or [[Cylindric function]]), |
||
| − | solution |
+ | solution \(f=f(z)\) of the Bessel equation |
| − | : |
+ | :\( \!\!\!\!\!\!\!\!\!\! (1) ~ ~ ~ \displaystyle |
| − | f''+f'/z+f=0 |
+ | f''+f'/z+f=0\) |
with integral representation |
with integral representation |
||
| − | : |
+ | : \(\!\!\!\!\!\!\!\!\!\!\!\!\!\! |
| − | \displaystyle Y_0(x)=\frac{-2}{\pi} \int_0^\infty \cos(x \cosh(t)) \mathrm d t |
+ | \displaystyle Y_0(x)=\frac{-2}{\pi} \int_0^\infty \cos(x \cosh(t)) \mathrm d t\) |
| − | valid at least for |
+ | valid at least for \(x>0\). |
| − | The [[explicit plot]] of |
+ | The [[explicit plot]] of \(Y_0\) of real argument is shown at the upper right corner, for comparison, \(J_0\) and \(J_1\) are also plotted; |
| − | the [[complex map]] of |
+ | the [[complex map]] of \(Y_0\) is shown below. |
==Various notations== |
==Various notations== |
||
| − | In the literture, and in particular, in the first editions of the tables by [[Granshtein,Ryzhik]], BesselY0 is called [[Neumann function]] and denoted |
+ | In the literture, and in particular, in the first editions of the tables by [[Granshtein,Ryzhik]], BesselY0 is called [[Neumann function]] and denoted \(N_0\). In the latest editions and in the tables by [[Abramowits,Stegun]], notation \(Y_0\) is used. |
<!-- |
<!-- |
||
more integral representations can be deduced. |
more integral representations can be deduced. |
||
| Line 31: | Line 31: | ||
</ref> |
</ref> |
||
| − | ==Relation to |
+ | ==Relation to \(J_0\)== |
| − | In the upper half–plane, id est, for |
+ | In the upper half–plane, id est, for \(\Re(z)>0\), the following relation with [[BesselJ0]] takes place: |
| − | : |
+ | : \(Y_0(z)=Y_0(-z)+2~ \mathrm i~ J_0(-z)\) |
| − | At |
+ | At \(\Re(z)<0\), the similar relation is valid: |
| − | : |
+ | : \(Y_0(z)=Y_0(-z)- 2~ \mathrm i~ J_0(-z)\) |
| − | These formulas are used for evaluation of |
+ | These formulas are used for evaluation of \(Y_0(z)\) at \(\Re(z)<0\) in the [[C++]] complex(double) implementation [[Bessely0.cin]] . |
==Behavior at zero== |
==Behavior at zero== |
||
| − | : |
+ | :\( \!\!\!\!\!\!\!\!\!\! (2) ~ ~ ~ \displaystyle |
Y_0(z)=\frac{2 (\log (z)+\gamma -\log (2))}{\pi |
Y_0(z)=\frac{2 (\log (z)+\gamma -\log (2))}{\pi |
||
}+\frac{z^2 (-\log (z)-\gamma +1+\log (2))}{2 |
}+\frac{z^2 (-\log (z)-\gamma +1+\log (2))}{2 |
||
\pi }+\frac{z^4 (2 \log (z)+2 \gamma -3-2 \log |
\pi }+\frac{z^4 (2 \log (z)+2 \gamma -3-2 \log |
||
(2))}{64 \pi }+O\left(z^6\right) |
(2))}{64 \pi }+O\left(z^6\right) |
||
| + | \) |
||
| − | $ |
||
| − | where |
+ | where \(~\gamma=\)[[EulerGamma]]\(~\approx 0.5772156649015329~\) is universal constant. |
==Expansion at large values of the argument== |
==Expansion at large values of the argument== |
||
| − | At large |
+ | At large \(|z|\), the expansion of \(Y_0\) can be written in analogy with that for \(J_0\): |
| − | : |
+ | :\( \!\!\!\!\!\!\!\!\!\!\!\! (3) ~ ~ ~ \displaystyle |
J_0(z)=\sqrt{\frac{2}{\pi z}} |
J_0(z)=\sqrt{\frac{2}{\pi z}} |
||
\left( \left(\frac{1}{8 z} -\frac{75}{1024 z^3}+O\left(\frac{1}{z^5}\right)\right) \sin \left(z-\frac{\pi }{4}\right) |
\left( \left(\frac{1}{8 z} -\frac{75}{1024 z^3}+O\left(\frac{1}{z^5}\right)\right) \sin \left(z-\frac{\pi }{4}\right) |
||
+\left(1-\frac{9}{128 z^2}+\frac{3675}{32768 z^4}+O\left(\frac{1}{z^6}\right)\right) \cos \left(z-\frac{\pi }{4}\right) |
+\left(1-\frac{9}{128 z^2}+\frac{3675}{32768 z^4}+O\left(\frac{1}{z^6}\right)\right) \cos \left(z-\frac{\pi }{4}\right) |
||
\right) |
\right) |
||
| + | \) |
||
| − | $ |
||
| − | : |
+ | :\( \!\!\!\!\!\!\!\!\!\!\!\! (4) ~ ~ ~ \displaystyle |
Y_0(z)=\sqrt{\frac{2}{\pi z}} |
Y_0(z)=\sqrt{\frac{2}{\pi z}} |
||
\left( \left(\frac{-1}{8 z}+\frac{75}{1024 z^3}+O\left(\frac{1}{z^5}\right)\right) \cos \left(z-\frac{\pi }{4}\right) |
\left( \left(\frac{-1}{8 z}+\frac{75}{1024 z^3}+O\left(\frac{1}{z^5}\right)\right) \cos \left(z-\frac{\pi }{4}\right) |
||
+\left(1-\frac{9}{128 z^2}+\frac{3675}{32768 z^4}+O\left(\frac{1}{z^6}\right)\right) \sin \left(z-\frac{\pi }{4}\right) |
+\left(1-\frac{9}{128 z^2}+\frac{3675}{32768 z^4}+O\left(\frac{1}{z^6}\right)\right) \sin \left(z-\frac{\pi }{4}\right) |
||
\right) |
\right) |
||
| + | \) |
||
| − | $ |
||
==Keywords== |
==Keywords== |
||
Latest revision as of 18:26, 30 July 2019
BesselY0, called also Neumann function, and also \(\mathrm{BesselY}_0\) or simply \(Y_0\) is kind of Bessel function (or Cylindric function), solution \(f=f(z)\) of the Bessel equation
- \( \!\!\!\!\!\!\!\!\!\! (1) ~ ~ ~ \displaystyle f''+f'/z+f=0\)
with integral representation
- \(\!\!\!\!\!\!\!\!\!\!\!\!\!\! \displaystyle Y_0(x)=\frac{-2}{\pi} \int_0^\infty \cos(x \cosh(t)) \mathrm d t\)
valid at least for \(x>0\).
The explicit plot of \(Y_0\) of real argument is shown at the upper right corner, for comparison, \(J_0\) and \(J_1\) are also plotted; the complex map of \(Y_0\) is shown below.
Contents
Various notations
In the literture, and in particular, in the first editions of the tables by Granshtein,Ryzhik, BesselY0 is called Neumann function and denoted \(N_0\). In the latest editions and in the tables by Abramowits,Stegun, notation \(Y_0\) is used. In Mathematica, BesselY0(z) can be accessed with BesselY[0,z] and is interpreted as "Bessel function of Second kind" [1]
Relation to \(J_0\)
In the upper half–plane, id est, for \(\Re(z)>0\), the following relation with BesselJ0 takes place:
- \(Y_0(z)=Y_0(-z)+2~ \mathrm i~ J_0(-z)\)
At \(\Re(z)<0\), the similar relation is valid:
- \(Y_0(z)=Y_0(-z)- 2~ \mathrm i~ J_0(-z)\)
These formulas are used for evaluation of \(Y_0(z)\) at \(\Re(z)<0\) in the C++ complex(double) implementation Bessely0.cin .
Behavior at zero
- \( \!\!\!\!\!\!\!\!\!\! (2) ~ ~ ~ \displaystyle Y_0(z)=\frac{2 (\log (z)+\gamma -\log (2))}{\pi }+\frac{z^2 (-\log (z)-\gamma +1+\log (2))}{2 \pi }+\frac{z^4 (2 \log (z)+2 \gamma -3-2 \log (2))}{64 \pi }+O\left(z^6\right) \)
where \(~\gamma=\)EulerGamma\(~\approx 0.5772156649015329~\) is universal constant.
Expansion at large values of the argument
At large \(|z|\), the expansion of \(Y_0\) can be written in analogy with that for \(J_0\):
- \( \!\!\!\!\!\!\!\!\!\!\!\! (3) ~ ~ ~ \displaystyle J_0(z)=\sqrt{\frac{2}{\pi z}} \left( \left(\frac{1}{8 z} -\frac{75}{1024 z^3}+O\left(\frac{1}{z^5}\right)\right) \sin \left(z-\frac{\pi }{4}\right) +\left(1-\frac{9}{128 z^2}+\frac{3675}{32768 z^4}+O\left(\frac{1}{z^6}\right)\right) \cos \left(z-\frac{\pi }{4}\right) \right) \)
- \( \!\!\!\!\!\!\!\!\!\!\!\! (4) ~ ~ ~ \displaystyle Y_0(z)=\sqrt{\frac{2}{\pi z}} \left( \left(\frac{-1}{8 z}+\frac{75}{1024 z^3}+O\left(\frac{1}{z^5}\right)\right) \cos \left(z-\frac{\pi }{4}\right) +\left(1-\frac{9}{128 z^2}+\frac{3675}{32768 z^4}+O\left(\frac{1}{z^6}\right)\right) \sin \left(z-\frac{\pi }{4}\right) \right) \)
Keywords
Bessel function BesselJ0 BesselJ1 BesselH0
References
- ↑ http://mathworld.wolfram.com/BesselFunctionoftheSecondKind.html Bessel Function of the Second Kind
