Difference between revisions of "Logistic operator"
| (4 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
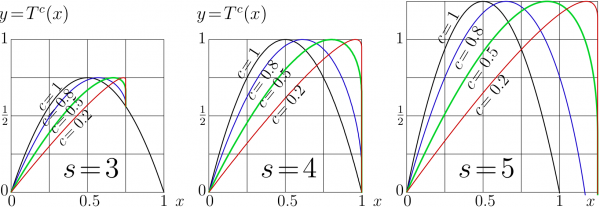
| − | [[File:Logi1a345T300.png|600px|thumb| Various iterates of |
+ | [[File:Logi1a345T300.png|600px|thumb|<small> Various iterates of \(T^c=\mathrm{LogisticOperator}_s\) for |
| − | + | \(s\!=\!3\) (left), \(s\!=\!4\) (center) and \(s\!=\!5\) (right) by eq.(6) from <ref name="logi"> |
|
| + | http://mizugadro.mydns.jp/PAPERS/2010logistie.pdf |
||
| ⚫ | |||
| + | D.Kouznetsov. Holomorphic extension of the logistic sequence. Moscow University Physics Bulletin, 2010, No.2, p.91-98. (Russian version: p.24-31) |
||
| ⚫ | |||
| + | </ref></small>]] |
||
| ⚫ | |||
| ⚫ | |||
| ⚫ | |||
| ⚫ | |||
==Logistic operator as transfer function== |
==Logistic operator as transfer function== |
||
| − | The [[explicit plot]]s of the Logistic operator for |
+ | The [[explicit plot]]s of the Logistic operator for \(s\!=\!3\), \(s\!=\!4\) and \(s\!=\!5\) are showm in pictures at right with black curves. |
| + | |||
| ⚫ | |||
| ⚫ | |||
| ⚫ | |||
| ⚫ | |||
| ⚫ | |||
| ⚫ | |||
| − | |||
==Superfunction of the LogisticOperator== |
==Superfunction of the LogisticOperator== |
||
| − | [[File:Logi5T1500.png|600px|thumb| |
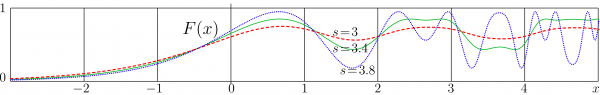
+ | [[File:Logi5T1500.png|600px|thumb|\(F(x)=\mathrm{LogisticSequence}_s(x)\) versus \(x\) for \(s\!=\!3\), \(s\!=\!3.4\), \(s\!=\!3.8\)]] |
| − | In [[TORI]], the LogisticOperator is used as an example of [[transfer function]] that allows construction of [[superfunction]] by [[regular iteration]] at the fixed point |
+ | In [[TORI]], the LogisticOperator is used as an example of [[transfer function]] that allows construction of [[superfunction]] by [[regular iteration]] at the fixed point \(L\!=\!0\). |
| − | The specific [[superfunction]] called [[LogisticSequence]] |
+ | The specific [[superfunction]] called [[LogisticSequence]]\(_s\) (without spacebar) refers to the solution \(F\) of the [[transfer equation]] |
| − | : |
+ | : \(\!\!\!\!\!\!\!\!\!\!\!(2) ~ ~ ~ ~ F(z\!+\!1)=T(F(z))\) |
| − | for the [[transfer function]] |
+ | for the [[transfer function]] \(T=\mathrm{LogisticOperator}_s\), constructed with [[regular iteration]] at this fixed point; it is assumed, that |
| − | : |
+ | : \(\!\!\!\!\!\!\!\!\!\!\!(3) ~ ~ ~ ~ F(z)=s^z+O(s^{2z})\). |
| − | Often, the argument |
+ | Often, the argument \(z\) is supposed to be a real number. In principle, it could be taken also from any set such that operations of multiplication (denoted with spacebar) and substraction (denoted with symbol "-" are somehow defined, but the properties of superfunctions in this case still need the additional research. In [[TORI]], at least up to year 2012, the LogisticOperator is used for complex variables |
(and not yet for [[quaternion]]s). |
(and not yet for [[quaternion]]s). |
||
| Line 30: | Line 33: | ||
==Abel function of the Logistic operator== |
==Abel function of the Logistic operator== |
||
| − | [[File:LogiarcT300.png|160px|thumb| |
+ | [[File:LogiarcT300.png|160px|thumb|\(\mathrm{ArcLogisticSequance}_s(x)\) versus \(x\) for various \(s\)]] |
The inverse finction of the LogisticSequence is called [[ArcLogisticSequence]]. It is plotted in figure at right. |
The inverse finction of the LogisticSequence is called [[ArcLogisticSequence]]. It is plotted in figure at right. |
||
| Line 36: | Line 39: | ||
this Abel function is considered as "principal", and all other as alternative. |
this Abel function is considered as "principal", and all other as alternative. |
||
| − | Function |
+ | Function \(G=\mathrm{ArcLogisticSequence}_s\) satisfies the [[Abel equation]] |
| − | :: |
+ | :: \(\!\!\!\!\!\!\!\!\!\!\!(4) ~ ~ ~ ~ G(T(z))=G(z)+1\) |
| − | for the [[transfer function]] |
+ | for the [[transfer function]] \(T=\mathrm{LogisticOperator}_s\). |
The efficient [[C++]] numerical implementations for funcitons [[LogisticSequence]] and [[ArcLogisticSequence]]; these implementations are available in the description of the pictures. |
The efficient [[C++]] numerical implementations for funcitons [[LogisticSequence]] and [[ArcLogisticSequence]]; these implementations are available in the description of the pictures. |
||
| Line 46: | Line 49: | ||
==Inverse function== |
==Inverse function== |
||
The inverse function of the Logistic operator can be expressed as follows <ref name="logi"> |
The inverse function of the Logistic operator can be expressed as follows <ref name="logi"> |
||
| − | http:// |
+ | http://mizugadro.mydns.jp/PAPERS/2010logistie.pdf |
D.Kouznetsov. Holomorphic extension of the logistic sequence. Moscow University Physics Bulletin, 2010, No.2, p.91-98. (Russian version: p.24-31) |
D.Kouznetsov. Holomorphic extension of the logistic sequence. Moscow University Physics Bulletin, 2010, No.2, p.91-98. (Russian version: p.24-31) |
||
</ref>: |
</ref>: |
||
| − | : |
+ | : \(\!\!\!\!\!\!\!\!\!\!\!(5) ~ ~ ~ ~\displaystyle \mathrm{LogisticOperator}_s^{\, -1}(z)= \frac{1}{2} - \sqrt{\frac{1}{4}-\frac{z}{s}}\) |
==Iteration of the Logistic operator== |
==Iteration of the Logistic operator== |
||
| − | For integer |
+ | For integer \(c\), the \(c\)th iteration of function \(T=\mathrm{LogisticOperator}_s\) can be evaluated by the straightforward application of \(T\) or \(T^{-1}=\mathrm{LogisticOperator}_s^{\, -1}\), repeated \(c\) or \(-c\) times: |
| − | : |
+ | : \(T^{-2}(z)=T^{-1}(T^{-1}(z))\) |
| − | : |
+ | : \(T^{-1}(z)=\mathrm{LogisticOperator}_s^{\, -1}(z)\) |
| − | : |
+ | : \(T^0(z)=z\) |
| − | : |
+ | : \(T^1(z)=T(z)=\mathrm{LogisticOperator}_s(z)\) |
| − | : |
+ | : \(T^2(z)=T(T(z))\) |
and so on. For non-integer values, the representation through the [[LogisticSequence]] is suggested; |
and so on. For non-integer values, the representation through the [[LogisticSequence]] is suggested; |
||
| − | : |
+ | : \(\!\!\!\!\!\!\!\!\!\!\!(6) ~ ~ ~ ~ T^c(z)=\mathrm{LogisticSequence}_s\Big( c + \mathrm{ArcLogisticSequence}_s(z)\Big)\) |
following the general rule of construction of iterations of a transfer function with specified [[Superfunction]] and [[Abel function]]. |
following the general rule of construction of iterations of a transfer function with specified [[Superfunction]] and [[Abel function]]. |
||
Properties of the superfunction [[LogisticSequence]] and the Abel function [[ArcLogisticSequence]] are known; the efficient algorithms for the evaluation are supplied. With these functions, the explicit plot of some iterations of the logistic operators are |
Properties of the superfunction [[LogisticSequence]] and the Abel function [[ArcLogisticSequence]] are known; the efficient algorithms for the evaluation are supplied. With these functions, the explicit plot of some iterations of the logistic operators are |
||
| Line 65: | Line 68: | ||
While the LogisticOperator is not monotonous function, the relation |
While the LogisticOperator is not monotonous function, the relation |
||
| − | : |
+ | : \(\!\!\!\!\!\!\!\!\!\!\!(6) ~ ~ ~ ~ \mathrm{LogisticOperator}_s^{\,c}\Big( \mathrm{LogisticOperator}_s^{\,d}(x) \Big)=\mathrm{LogisticOperator}_s^{\,c+d}(x)\) |
| − | is valid only for moderate values of |
+ | is valid only for moderate values of \(x\), that should not exceed the branchpoint of function \(\mathrm{ArcLogisticSequence}_s\). For various s, these brancpoints are shown with black circles in figure at right. |
==References== |
==References== |
||
| Line 74: | Line 77: | ||
[[LogisticSequence]], [[Logistic sequence]], [[Transfer function]] |
[[LogisticSequence]], [[Logistic sequence]], [[Transfer function]] |
||
| + | |||
| + | [[Category:Logistic operator]] |
||
| + | [[Category:Transfer function]] |
||
| + | [[Category:Iteration]] |
||
Latest revision as of 19:54, 5 August 2020
Logistic operator (or LogisticOperator) is quadratic function of specific kind determined with the single parameter \(s\), which is usulally assumed to be real, \(s\!>\!1\):
- \(\!\!\!\!\!\!\!\!\!\!\!(1) ~ ~ ~ ~ \mathrm{LogisticOperator}_s(z) = s\,z \,(1\!-\!z)\)
(Parameter \(s\) is often denoted also with letters \(u\) or \(q\)). In such a way, \(\mathrm{LogisticOperator}_s\) is entire function.
Logistic operator as transfer function
The explicit plots of the Logistic operator for \(s\!=\!3\), \(s\!=\!4\) and \(s\!=\!5\) are showm in pictures at right with black curves.
The LogisticOperator is interpreted as transfer function; one of corresponding superfunctions is called Logistic sequence, and the corresponding Abel function is called ArcLogisticSequence.
They are built up with regular iteration at the fixed point \(L\!=\!0\).
These superfunction and abelfunction are used to plot the non-integer iterates of the LogisticOperator; these iterates are shown with colored curves in figure above.
Superfunction of the LogisticOperator
In TORI, the LogisticOperator is used as an example of transfer function that allows construction of superfunction by regular iteration at the fixed point \(L\!=\!0\). The specific superfunction called LogisticSequence\(_s\) (without spacebar) refers to the solution \(F\) of the transfer equation
- \(\!\!\!\!\!\!\!\!\!\!\!(2) ~ ~ ~ ~ F(z\!+\!1)=T(F(z))\)
for the transfer function \(T=\mathrm{LogisticOperator}_s\), constructed with regular iteration at this fixed point; it is assumed, that
- \(\!\!\!\!\!\!\!\!\!\!\!(3) ~ ~ ~ ~ F(z)=s^z+O(s^{2z})\).
Often, the argument \(z\) is supposed to be a real number. In principle, it could be taken also from any set such that operations of multiplication (denoted with spacebar) and substraction (denoted with symbol "-" are somehow defined, but the properties of superfunctions in this case still need the additional research. In TORI, at least up to year 2012, the LogisticOperator is used for complex variables (and not yet for quaternions).
In principle, other superfunctions of the LogisticOperator can be constructed in the similar way. That called LogisticSequance seems to be the simplest one. According to the Axiom TORI number 6, this superfunction is considered as "principal", and all others superfunctions of the LogisticOperator are treated as alternatives.
Abel function of the Logistic operator
The inverse finction of the LogisticSequence is called ArcLogisticSequence. It is plotted in figure at right. Many superfuncitons for the given transfer function exist; and many Abel functions exist too. That called ArcLogisticSequance seems to be the simplest one. According to the Axiom TORI number 6, this Abel function is considered as "principal", and all other as alternative.
Function \(G=\mathrm{ArcLogisticSequence}_s\) satisfies the Abel equation
- \(\!\!\!\!\!\!\!\!\!\!\!(4) ~ ~ ~ ~ G(T(z))=G(z)+1\)
for the transfer function \(T=\mathrm{LogisticOperator}_s\).
The efficient C++ numerical implementations for funcitons LogisticSequence and ArcLogisticSequence; these implementations are available in the description of the pictures.
Inverse function
The inverse function of the Logistic operator can be expressed as follows [1]:
- \(\!\!\!\!\!\!\!\!\!\!\!(5) ~ ~ ~ ~\displaystyle \mathrm{LogisticOperator}_s^{\, -1}(z)= \frac{1}{2} - \sqrt{\frac{1}{4}-\frac{z}{s}}\)
Iteration of the Logistic operator
For integer \(c\), the \(c\)th iteration of function \(T=\mathrm{LogisticOperator}_s\) can be evaluated by the straightforward application of \(T\) or \(T^{-1}=\mathrm{LogisticOperator}_s^{\, -1}\), repeated \(c\) or \(-c\) times:
- \(T^{-2}(z)=T^{-1}(T^{-1}(z))\)
- \(T^{-1}(z)=\mathrm{LogisticOperator}_s^{\, -1}(z)\)
- \(T^0(z)=z\)
- \(T^1(z)=T(z)=\mathrm{LogisticOperator}_s(z)\)
- \(T^2(z)=T(T(z))\)
and so on. For non-integer values, the representation through the LogisticSequence is suggested;
- \(\!\!\!\!\!\!\!\!\!\!\!(6) ~ ~ ~ ~ T^c(z)=\mathrm{LogisticSequence}_s\Big( c + \mathrm{ArcLogisticSequence}_s(z)\Big)\)
following the general rule of construction of iterations of a transfer function with specified Superfunction and Abel function. Properties of the superfunction LogisticSequence and the Abel function ArcLogisticSequence are known; the efficient algorithms for the evaluation are supplied. With these functions, the explicit plot of some iterations of the logistic operators are shown in the figure.
While the LogisticOperator is not monotonous function, the relation
- \(\!\!\!\!\!\!\!\!\!\!\!(6) ~ ~ ~ ~ \mathrm{LogisticOperator}_s^{\,c}\Big( \mathrm{LogisticOperator}_s^{\,d}(x) \Big)=\mathrm{LogisticOperator}_s^{\,c+d}(x)\)
is valid only for moderate values of \(x\), that should not exceed the branchpoint of function \(\mathrm{ArcLogisticSequence}_s\). For various s, these brancpoints are shown with black circles in figure at right.
References
- ↑ 1.0 1.1 http://mizugadro.mydns.jp/PAPERS/2010logistie.pdf D.Kouznetsov. Holomorphic extension of the logistic sequence. Moscow University Physics Bulletin, 2010, No.2, p.91-98. (Russian version: p.24-31)