Difference between revisions of "AbelFactorial"
m (→Regular iteration: prime) |
(mizugadro) |
||
| Line 21: | Line 21: | ||
==Regular iteration== |
==Regular iteration== |
||
For the evaluation of AbelFactorial, the [[regular iteration]] at the fixed point 2 of factorial is efficient. The following expansion is suggested <ref name="fac"> |
For the evaluation of AbelFactorial, the [[regular iteration]] at the fixed point 2 of factorial is efficient. The following expansion is suggested <ref name="fac"> |
||
| − | http:// |
+ | http://mizugadro.mydns.jp/PAPERS/2010superfae.pdf |
D.Kouznetsov, H.Trappmann. Superfunctions and square root of factorial. Moscow University Physics Bulletin, 2010, v.65, No.1, p.6-12. |
D.Kouznetsov, H.Trappmann. Superfunctions and square root of factorial. Moscow University Physics Bulletin, 2010, v.65, No.1, p.6-12. |
||
<!-- (Russian version: p.8-14) |
<!-- (Russian version: p.8-14) |
||
| Line 57: | Line 57: | ||
==Previous notation== |
==Previous notation== |
||
In publication <ref name="fac"> |
In publication <ref name="fac"> |
||
| − | http:// |
+ | http://mizugadro.mydns.jp/PAPERS/2010superfae.pdf |
D.Kouznetsov, H.Trappmann. Superfunctions and square root of factorial. Moscow University Physics Bulletin, 2010, v.65, No.1, p.6-12.</ref>, |
D.Kouznetsov, H.Trappmann. Superfunctions and square root of factorial. Moscow University Physics Bulletin, 2010, v.65, No.1, p.6-12.</ref>, |
||
function $\tilde G$ is called "ArcSuperFactorial" and denoted with letter $G$; however, it is more convenient to deal with a function that takes integer values at least for some integer values of the argument; therefore the representation (5) is recommended. |
function $\tilde G$ is called "ArcSuperFactorial" and denoted with letter $G$; however, it is more convenient to deal with a function that takes integer values at least for some integer values of the argument; therefore the representation (5) is recommended. |
||
| Line 72: | Line 72: | ||
and at $c=1/2$, such a representation determines the square root of factorial, id est, $\sqrt{!\,}$, that is used as Logo of the Physics Department of the Moscow State University and part of logo of [[TORI]] shown in the left top corner of each page of TORI. In some facility of the half-line $z\!>\!2$, the following relation holds |
and at $c=1/2$, such a representation determines the square root of factorial, id est, $\sqrt{!\,}$, that is used as Logo of the Physics Department of the Moscow State University and part of logo of [[TORI]] shown in the left top corner of each page of TORI. In some facility of the half-line $z\!>\!2$, the following relation holds |
||
<ref name="suomi2011"> |
<ref name="suomi2011"> |
||
| − | http:// |
+ | http://mizugadro.mydns.jp/PDF/2011suomi.pdf |
D.Kouznetsov. NON-INTEGER ITERATES OF ANALYTIC FUNCTIONS. Slideshow presented at [[FMI]], Finland, 2011.06.15 |
D.Kouznetsov. NON-INTEGER ITERATES OF ANALYTIC FUNCTIONS. Slideshow presented at [[FMI]], Finland, 2011.06.15 |
||
</ref> |
</ref> |
||
Revision as of 18:28, 25 September 2013
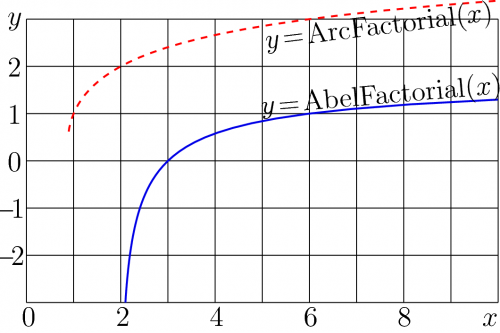
AbelFactorial is holomorphic function, inverse of the SuperFactorial, Abel function of Factorial constructed with regular iteration at its fixed points 2 in such a way that
- $\mathrm{AbelFactorial}(z^*)=\mathrm{AbelFactorial}(z)^*$.
- $\mathrm{AbelFactorial}(3)=0$
- $\displaystyle
\lim_{x\rightarrow -\infty}\mathrm{AbelFactorial}(x+\mathrm i y)=2 ~ ~ \forall y \in \mathbb R: y\!\ne\!0$
Along the real axis, AbelFactorial is slowly growing function, its growth is much slower than that of ArcFactorial shown in the top figure at right.
ArcFactorial is analytic in the complex plane with cut in the direction of the negative part f the real axis; the range of holomorphizm is $\mathbb C \backslash \{ x\in \mathbb{R}: x\le 2\}$.
Abel equation
AbelFactorial $G$ satisfies the Abel equation
- (1) $~ ~ ~ G(z!)=G(z)+1$
The Abel equation is consistent with the transfer equation for the SuperFactorial $F=G^{-1}$:
- (2) $~ ~ ~ \mathrm{Factorial}(F(z))=F(z\!+\!1)$
Regular iteration
For the evaluation of AbelFactorial, the regular iteration at the fixed point 2 of factorial is efficient. The following expansion is suggested [1]:
- (2) $~ ~ ~
\tilde G(z)= \frac{1}{k} \log\!\left(\sum_{n=1}^{N-1}U_{n}(z\!-\!2)^{n}+\mathcal{O}(z\!-\!2)^N\right) $ where
- (3) $~ ~ ~ k=\ln\!\big(3+2\!~\mathrm{Factorial}^{\prime}(0)\big)=\ln(3-2\!~\gamma)
\approx 0.6127874523307$, $\gamma$ is the Euler's constant;
- $U_1 =1$
- $U_{2}=-\frac{\pi^2+6\gamma^{2}-18\gamma+6}{12(3-5\gamma+2\gamma^{2})}
\approx 0.798731835$
The coefficients $U$ can be found substituting the representation (2) into the Abel equation (1) and expanding the result to the power series with small parameter $z\!-\!2$.
Extension of the regular iteration
If $|z\!-\!2|$ is not small, then the representation
- (4) $~ ~ ~ \tilde G(z)=\tilde G (\mathrm{ArcFactorial}^n(z))+n$
can be be used for integer $n$.
The AbelFactorial $G$ can be expressed through $\tilde{G}$;
- (5) $~ ~ ~ G(z)=\tilde G(z) - \tilde G(3)$
in such a way that $G(3)=0$, corresponding to $\mathrm{SuperFactorial}(0)=3$.
Previous notation
In publication [1], function $\tilde G$ is called "ArcSuperFactorial" and denoted with letter $G$; however, it is more convenient to deal with a function that takes integer values at least for some integer values of the argument; therefore the representation (5) is recommended.
Iteration of Factorial
Together with Superfactorial $F=G^{-1}$, the AbelFactorial $G$ allow to express the $c$-th iteration of Factorial as follows:
- (6) $~ ~ ~
\mathrm{Factorial}^c(z)= F(c+G(z))$
where $c$ has no need to be integer. In particular,
at $c=1$, $~ \mathrm{Factorial}^c(z)=z!$;
at $c=0$, $~ \mathrm{Factorial}^c(z)=z$;
at $c=-1$, $~ \mathrm{Factorial}^c(z)=\mathrm{ArcFactorial}(z)$;
and at $c=1/2$, such a representation determines the square root of factorial, id est, $\sqrt{!\,}$, that is used as Logo of the Physics Department of the Moscow State University and part of logo of TORI shown in the left top corner of each page of TORI. In some facility of the half-line $z\!>\!2$, the following relation holds
[2]
- (7) $~ ~ ~
\sqrt{!\,} \Big( \sqrt{!\,}\big(z\big)\Big)= z! $
References
- ↑ 1.0 1.1
http://mizugadro.mydns.jp/PAPERS/2010superfae.pdf
D.Kouznetsov, H.Trappmann. Superfunctions and square root of factorial. Moscow University Physics Bulletin, 2010, v.65, No.1, p.6-12.
Cite error: Invalid
<ref>tag; name "fac" defined multiple times with different content - ↑ http://mizugadro.mydns.jp/PDF/2011suomi.pdf D.Kouznetsov. NON-INTEGER ITERATES OF ANALYTIC FUNCTIONS. Slideshow presented at FMI, Finland, 2011.06.15