Shoka function

\(u\!+\!\mathrm i v = \mathrm{Shoka}(x\!+\mathrm i y)\)

Shoka function is holomorphic function,

\( \mathrm{Shoka}(z)=z+\ln\!\Big( \exp(-z) +\mathrm e -1\Big)\)

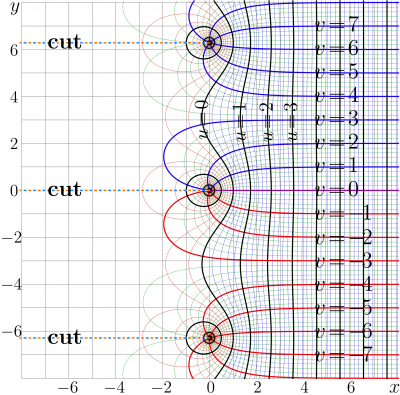
Complex map of the Shoka function is shown in figure at right.

Range of holomorphizm

The Shoka function is holomorphic at the complex plane with cuts

\( x+ (1\!+\!2n) \mathrm i \pi ~\) at real \(x\) and integer \(n\).

In such a way, the countable set of cut lines are directed to the left.

Inverse function

\(u\!+\!\mathrm i v= \mathrm{ArcShoka}(x\!+\!\mathrm i y)\)

The inverse function \(\mathrm{ArcShoka}=\mathrm{Shoka}^{-1}\) can be expressed as elementary function; for \(|\Im(z)| < \pi\),

\(\displaystyle \mathrm{ArcShoka}(z)= z + \ln \!\left( \frac{1\!-\!\mathrm e^{-z}}{\mathrm e \!-\!1} \right)\)

Some properties of the inverse function are collected in the special article ArcShoka.

Complex maps of Shoka and ArcShoka look similar. The following relation takes place:

\(\mathrm{ArcShoka}(z)=\mathrm{Shoka}\big(z-\ln(\mathrm e\! -\!1) - \mathrm i \pi\big) -\ln(\mathrm e\!-\!1) +\mathrm i \pi\)

that can be verified with the direct substitution.

Shoko function

In vicinity of the real axis, the Shoka function coincides with the Shoko function,

\( \mathrm{Shoko}(z) = \ln\!\Big( 1+ \exp(z)(\mathrm e -1)\Big)\)

However, the cut lines of the Shoko function are directed to the right, and the Shoko function is periodic with period \(P=2 \pi \mathrm i\).

Applications in Laser science

As the Shoko function, the Shoka function is superfunction of the Keller function and describes the evolution of the short pulse of light in a two-level laser medium. Properties and expansions of the Shoka function are similar to those of the Shoko function and are not repeated here.

Tania function

The Shoka function behaves similar to the Tania function, but have countable set of cutlines in the left hand side of the complex plane, while the Tania function has only two cut lines.

Origin of the name

Initially, the Shoko function had been implemented; that function is named after Shoko san. Then in happened, that the cut lines of the Shoko function go to the right hand side direction, that makes difficult its comparison with the Tania function in the complex plane. Name Shoka function is created as small modification of name Shoko function.

Keywords

Shoko function, Keller function, Superfunction, Laser science