Maps of tetration

\(y\!=\!\mathrm{tet}_b(x)\) versus \(x\) for various \(b\); plot from book

Base \(b=\sqrt{2}\approx 1.41\)]]

Henryk base, \(b=\exp(1/\mathrm e)\approx 1.44\)

Base \(b=1.5\)

Binary tetration, \(b=2\)

Natural base, \(b=\mathrm e \approx 2.71\)

Sheldon base, \(b=1.52598338517+0.0178411853321 \,\mathrm i\)

Article Maps of tetration collects some explicit plots and complex maps of tetration \(\mathrm{tet}_b\) to various values of base \(b\).

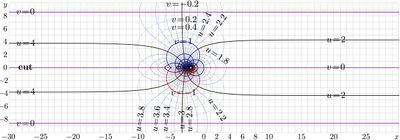
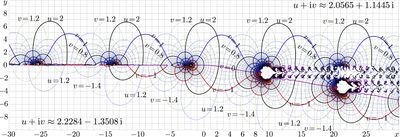
For real values of base \(b\), the real-real plots \(y\!=\!\mathrm{tet}_b(x)\) are shown in the upper figure at right.

Below, the complex maps are shown. The following cases are represented:

\(b=\sqrt{2} \approx 1.41\)
\(b=\exp(1/\mathrm e) \approx 1.44\)
\(b=1.5\)
\(b=2\)
\(b=\mathrm e \approx 2.71\)
\(b=1.52598338517+0.0178411853321 \,\mathrm i\)

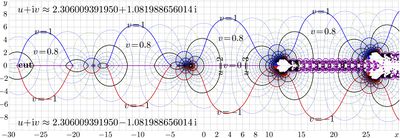
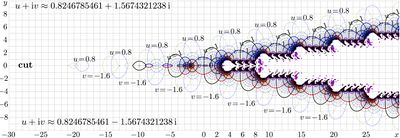
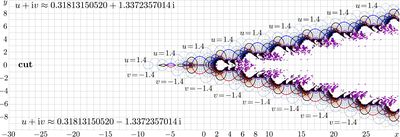
Tetration tet is shown with lines of constant real part \(u\) and lines of constant imaginary part \(v\); \(u\!+\!\mathrm i v=\mathrm {tet}_b(x\!+\!\mathrm i y)\)

b=sqrt(2)

For this case, the regular iteration at fixed point \(L=2\) is used. The evaluation is described in the Mathematics of Computation .

b= e^(1/e)   (approximately 1.44)

For \(b=\exp(1/\mathrm e)\approx 1.44\), the exotic iteration at fixed point \(L=\mathrm e\approx 2.71\) is used. The evaluation is described at the Mathematics of Computation , 2012.

b > e^(1/e)

For \(b>\exp(1/\mathrm e)\approx 1.44\), the Cauchi integral is used for evaluation. It is described in Mathematics of Computation .

Historically, evaluation for the case \(b=\mathrm e\) was first to be reported. Namely for this case, the special code fsexp.cin is loaded; it is described in Vladikavkaz Mathematical Jorunal .

The same algorithm can be used also for other values of base; in particular, for \(b=1.5\) and for \(b=2\); these cases are shown in figures at right.

Sheldon base b=1.52598338517+0.0178411853321 i

Tetration to Sheldon base \(b\!=\!1.52598338517\!+\!0.0178411853321\mathrm i\) is considered by the special request from Sheldon Levenstein. For this base, tetration was believed to be especially difficult to evaluate.

The evaluation uses almost the same algorithm of the Cauchi integral .

The small modification had been applied to the original algorithm; the condition \(F(z^*)=F(z)^*\) is suppressed at the numerical solving of the corresponding integral equation for values o superfunction along \(\Im(z)=\mathrm{const}\). No difficulties, specific namely for this complex value of base \(b\), had been revealed.

Book

The maps are plotted with the conto.cin code in C++. The Latex codes are used to add the labels. All the maps at right are supplied with generators; the colleagues may download the code and reproduce them. If some generator does not work as expected, let me know and let us correct it.

The algorithms, used to evaluate the tetration, are described in Book «Superfunctions» , 2020
and also in the Russian version «Суперфункции» , 2014.

Reuse

The explicit plot and the complex maps above are supplied with the generators.

The colleagues are invited to download these generators, to reproduce the pictures and to modify them adopting for the new needs.

At the reuse, please, attribute the source and indicate the modification, if any.
This helps to trace the bugs, if any.

2016-2018, William Paulsen and Samuel Cowgill suggest an alternative algorithm for evaluation of tetration to general base \(b\) . These results are not supplied with the C++ implementations and not represented here.

References

Keywords

«Ackermann», «Complex map», «Superfunction», «Superfunctions», «Tetration»,

«Суперфункции»,