SdPow
SdPow is specific superpower function, id est, the superfunction of the power function \(~z\mapsto z^a\!=\!\exp(\ln(z) \,a)~\)
For given parameter \(a\),
\(\mathrm{SdPow}_a(z)=\exp(a^z)\)
Usually, it is assumed, that \(a\!>\!1\).
Transfer function
Function \(F\!=\!\mathrm{SdPow}_a\) is superfunction for the specific power function \(T(z)\!=\!z^a\). The superfunction satisfies the transfer equation
\(T(F(z))=F(z\!+\!1)\)
For this specific transfer function \(T\), the two real-holomorphix solutions are SuPow and SdPow:
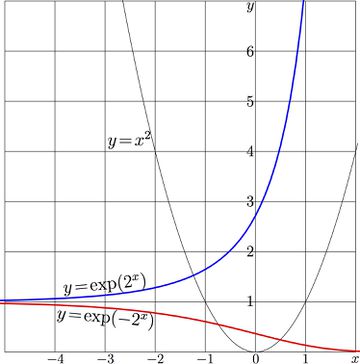
\(\mathrm{SdPow}_a(z)=\exp(-a^z)\)
\(\mathrm{SuPow}_a(z)=\exp(a^z)\)
For the power function both, the superfunctions and the Abel functions can be expressed as elementary functions. For \(a\!=\!2\), these functions are who functions are shown in Fig.1.
For the same \(a\!=\!2\), the complex map of function SdPow is shown in FIg.2.
References
Keywords
AdPow, Elementary function, Power function, SdPow, SuPow, Superfunction Superpower
